Surface Area of a Cylinder Calculator

This surface area of a cylinder calculator is a handy tool that quickly finds all of the three types of surface areas:

• The base surface area of a cylinder;
• The lateral surface area of a cylinder; and
• The total surface area of a cylinder.

Keep reading if you want to learn what is the surface area of a cylinder formula and how to find the surface area of a cylinder. You may also want to estimate other parameters of a cylinder – just check out our right cylinder calculator. You'll become a cylinder expert in no time!

A cylinder is a three-dimensional solid limited by two congruent circular surfaces (bases) and one lateral surface. Although cylinders may take many forms, the term cylinder usually means the right circular cylinder. Our surface area of a cylinder calculator is dedicated to this type of cylinder. The cylinder is right when one of its bases lies exactly above the other base and oblique if it doesn't. Generalized cylinders can have any plain, closed surface as their base.

To estimate the surface area of a cylinder, you need to visualize it as a net. It is as if you open the cylinder just like a carton box and flatten it out. Use your imagination! And what will you get? The answer is that a right circular cylinder consists of two circles and one rectangle, as you can see in the figure below.

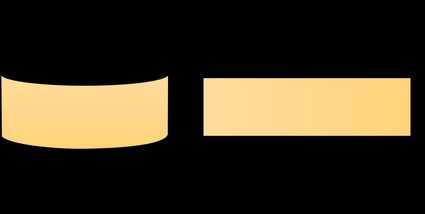
Therefore, the base surface area of a cylinder equals two times the area of a circle with the radius r, and the lateral surface area of a cylinder is the area of a rectangle. The first side of this rectangle is the height of the cylinder h, and the second is the circumference of the base equal to 2 × π × r, according to the circumference calculator.

Now that we know how to find the surface area of a cylinder let's derive appropriate formulas for the surface area of a right circular cylinder. To calculate the base surface area, you need to compute the area of a circle with the radius r. But remember that every cylinder has two bases! Thus, you need to multiply it by two:

base_area = 2 × π × r²

Estimation of the lateral surface area is even easier. Because the area of a rectangle is the product of its sides, we can write that:

lateral_area = (2 × π × r) × h,

where

• 2 × π × r is the circumference of the base circle,
• h is the height of a cylinder.

Finally, the total surface area of the cylinder formula is simply the sum of the base surface area and the lateral surface area:

total_area = base_area + lateral_area,

or total_area = 2 × π × r² + (2 × π × r) × h,

or total_area = 2 × π × r × (r + h).

With the surface area of a cylinder calculator, you can perform all the calculations in many different units. If you want to learn more about area unit conversion, check out our area converter now!

While you can also use this tool to calculate the volume of a cylinder, we also have a dedicated tool called cylinder volume calculator.

Let's solve some example problems with the surface area of a cylinder calculator.

1. Question: What is the surface area of a cylinder with the base radius r = 2 cm and the height h = 3 cm?

   • Answer: The base surface area equals 25.133 cm², the lateral surface area equals 37.7 cm², and the total surface area equals 62.83 cm².

2. Question: What is the surface area of a cylinder with a base diameter d = 10 cm and height h = 5 cm?

   • Answer: Firstly, you need to divide the diameter by two to estimate the radius of the circle using the formula r = d/2 = 5 cm or use another tool: the circle calc: find r. Then enter it, together with the height, into the empty fields of our calculator. In this problem, the base surface area equals 157.08 cm², the lateral surface area equals 157.08 cm², and the total surface area is 314.16 cm².

3. Question: What is the height of a cylinder with a total surface area of 200 cm² and radius r = 2 cm?

   • Answer: You can use our surface area of a cylinder calculator in this case too! Just enter the above values, and you will find that the height equals 13.915 cm.