Frequency Calculator

The frequency calculator will let you find a wave's frequency given its period or its wavelength and velocity in no time. You can choose a wave velocity from the preset list, so you don't have to remember.

In the text, you'll also find the frequency definition, two frequency formulas (period to frequency and wavelength to frequency), and a few examples showing how to calculate frequency.

If you want to know more about waves, check the wavelength calculator, and if you want to know the frequency of musical notes, check out the note frequency calculator.

Have a look at the following model of a wave; it will help you understand the terms used in the frequency definition below it.

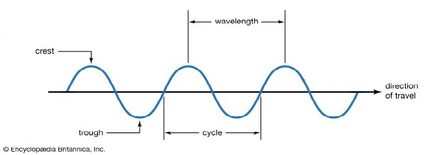
Frequency is the number of completed wave cycles per second. In other words, frequency tells us how many wave crests pass a given point in a second.

This frequency definition leads us to the simplest frequency formula:

$f$ denotes frequency, and $T$ stands for the time it takes to complete one wave cycle measured in seconds.

The SI frequency unit is Hertz (Hz), which equals 1/s (one cycle per second). Other frequency units include millihertz (mHz), kilohertz (kHz), megahertz (MHz), gigahertz (GHz), and terahertz (THz).

Have a look at another picture which will allow us to see the connection between frequency and wavelength. Wavelength is the distance between two adjacent crests (or troughs). In other words - it is the length of one wave cycle. The longer the wavelength, the lower the frequency:

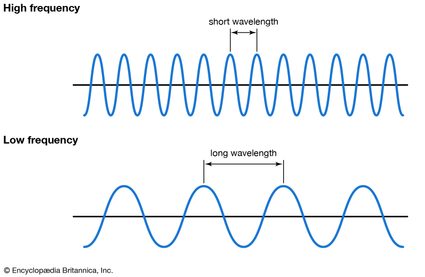
Another fact we need - how fast the waves travel at (wave velocity) determines how many of them will pass a given point per second. This means the higher the wave velocity, the higher the frequency.

These two relationships between frequency and wavelength ($\lambda$) and between frequency and velocity ($v$) bring us to the following frequency equation:

Our frequency calculator incorporates the above-mentioned frequency formulas. Therefore, you can use it as a period to frequency calculator or a wavelength to frequency calculator.

How to use it as a period to frequency calculator (How to find frequency if you know period)

1. Input the time in which one wave cycle occurs (period). The calculator will determine the frequency.

2. You can use this calculator to determine the period if you know its frequency.

How to use it as a wavelength to frequency calculator (How to calculate frequency from wavelength)

1. Choose the type of wave and its medium from the list in the first field. By default, we have this set to light in a vacuum. If you select the "Set a custom wave velocity" option, you can enter a wave velocity of your choice.

2. Enter the wavelength, and the frequency value will appear.

So, basically, you can enter any two variables, and the third will appear immediately 😀

To resolve any doubts about how to calculate frequency from the period, let's analyze some simple examples. Firstly, recall the frequency equation:

Example 1:

How to find the frequency of a wave in which one cycle is completed in 0.25s:

Remember to convert $\frac{1}{\text{s}}$ to the frequency unit:

Example 2:

How to find the frequency of a wave in which 360 cycles occur in 1 minute:

This time, we want to learn how to find the frequency of a wave if you're given the wave velocity and wavelength. You need to use the following frequency formula:

Example 1:

A wave's velocity equals 320 m/s, and its wavelength is 8 m. Finding its frequency, we have:

Example 2:

Find the frequency of light if wavelength equals 3,000 km. The wave velocity equals the speed of light in vacuum approximately equal to $300,\hspace{-0.05cm}000\ \tfrac{\text{km}}{\text{s}}$. Using the frequency formula, we get:

In wireless communication, we have an ellipsoid volume between the transmitter antenna and the receiver antenna. This region is determined by the distance between the antennas and the frequency of the wireless wave. It is called Fresnel Zone and looks like this:

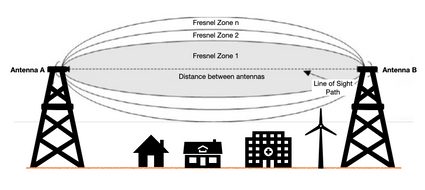
We infer from the main Fresnel zone formula that the higher the frequency, the smaller the ellipsoid volume required to be free for proper wireless communication. Contrarily, for a low frequency, we get a larger Fresnel zone which buildings or trees might easily block and, thus, produce unstable wireless communication.

When two waves of different frequencies interfere with each other constructively and destructively, they produce an interaction pattern called a beat. You can find the specific frequency of a beat with Omni's beat frequency calculator.

Another interesting phenomenon due to the interaction of waves with different frequencies is resonance. Resonance can occur, for example, when air passes through a cavity, such as when blowing the neck of an empty bottle.

This resonant cavity is known as a Helmholtz resonator. If the volume of the cavity changes, the resonant frequency changes. You can determine the resonance frequency of cavities with different volumes using our Helmholtz resonator calculator.