Rectangle Diagonal Angle Calculator

Our rectangle diagonal angle calculator is a great tool to help you to calculate the angles created when we draw diagonal lines through a rectangle. Whether you are studying geometry or just doing research for a project, we have what you need. Here we will discuss:

• What is the meaning of the diagonal of a rectangle.
• How many diagonals does a rectangle have?
• The formulas used by our rectangle diagonal angle calculator.
• Some mathematical facts related to finding the diagonals of a rectangle.

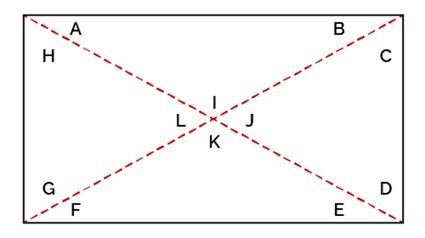
A diagonal of a rectangle is a straight line drawn through a rectangle that connects the vertices that are not adjacent. Each rectangle has two diagonals. The diagonals of a rectangle bisect each other.

Now that you understand what diagonals mean, here are a few facts that will help us to find the diagonal angles of a rectangle:

• When we draw two diagonal lines through a rectangle, they form two pairs of congruent angles at their point of intersection.
• The congruent angles are the ones opposite to each other. The angles opposite the intersection are also duplicates of each other.
• The vertices of a rectangle form angles of 90°.
• Linear angles, e.g., I and J in the diagram, add up to 180°.
• The interior angles of a triangle also add up to 180°.

These bits of information are crucial to finding the angles formed by the diagonals when we know the values of one side. Discovering the others is pretty simple if you know any other interior angles. Our rectangle diagonal angle calculator uses the following formulas to find the angles formed by the diagonals:

1. 180° = A + B + I
2. A = B = F = E
3. 90° = A + H
4. I = K
5. L = J
6. H = G = C = D

So if we are given A = 46° then B, F and E will also be equal to 46°
Since A + B + I = 180°:

I = 180° - (A+B) = 180° - (46° + 46°)
I = 180° - 92°
I = 88°

So K is also 88°.

Because we know that A + H = 90° and A = 46° then:

H = 90° - 46° = 37°

Therefore G, C and D = 37°.

Given I + J = 180°:

J = 180° - 88° = 92°

And since J = L:

L = 92°

At Omni, we have tons of calculators to meet your needs. Here are a few to help you to calculate values related to geometric shapes:

• Area of a rectangle calculator
• Dimensions of a rectangle calculator
• Diagonal of a rectangle calculator
• Perimeter of a rectangle
• Surface area of a rectangle calculator
• Length of a rectangle calculator
• Width of a rectangle calculator
• Length and width of rectangle given area calculator
• Length and width of rectangle given perimeter calculator
• Perimeter of a rectangle with given area
• Rectangle scale factor calculator
• Square feet of a rectangle calculator
• Golden rectangle calculator