Snowman Calculator
This ultimate snowman calculator uses science-based rules to help you build the perfect snowman. Not sure if there is enough snow for the snowman of your dreams? Or instead, planning to turn all that snow in your yard into the biggest snowman possible and wondering how tall it'll end up being? Apart from Omni's snowman calculator and a yard full of snow, you'll only need several lumps of black coal, a carrot, a hat, and a scarf. Are you ready for a very cool adventure? Let's go!
Snowmen-building phenomenon
It's hard to comprehend, really. Each year thousands upon thousands of snowmen, varying in sizes and shapes, appear throughout regions blessed with even the humblest of snowfalls. It's no trivial matter: there's more than 89,000 YouTube videos that offer tips and instructions on how to build better snowmen, and in 2011 a US patent was granted for Apparatus for facilitating the construction of a snow man/woman ().
Everybody realizes there are lots and lots of factors that are significant when calling snowmen to life. Some have to do with the type of snow available (its dryness/wetness, density, purity, freshness) and the temperature outside, others with the size & shape of the snowman: the number of balls constituting the snowman, their diameters, ratios, and so on. Does it mean you have to be an academic-level expert in 3D geometry, thermodynamics, crystallography, meteorology, etc., to build the perfect snowman (or, at least, a better one than that of your neighbors)? Don't worry! Fortunately, it suffices to use our perfect snowman calculator.
And when the snow is over and it's time to return to exams and school, use our grade calculator and test grade calculator to calculate your results!
How to use this snowman calculator?
-
Choose a mode of the calculator. There are two of them, and they help you:
-
Estimate how big a snowman you can build with the snow accumulated in your yard.
-
Decide how much snow is required for a snowman of a particular size and shape.
-
-
Enter the data, depending on the mode you've chosen:
-
For the first mode, type in the thickness of snow cover and the size of your yard. If the yard is roughly rectangular or circular, you may alternatively enter its width & length or radius.
-
For the other mode, enter the size and shape of the snowman of your dreams. There are several pre-set shape propositions and the custom option as well!
-
-
The snowman calculator gives you the answers in the blink of an eye. Tip: to turn the required snow volume into the snow area, tell us how thick the snow cover in your yard is.
-
Additionally, our calculator returns the weights of the snowman's parts so that you won't end up with balls too heavy to lift.
-
If you want to know the approximate lifetime of your snowman, enter the snow temperature and the air temperature.
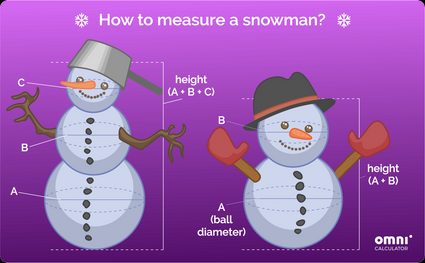
Optimal snowman shape. Why is it always spheres?
Have you ever wondered why the overwhelming majority of snowmen consists of snowy spheres and not, for instance, cubes nor cylinders? Sure, spheres are fairly easy to be made by exploiting the, well, snowball effect: you start small and push the ball around collecting more and more snow. The most important reason, however, is mathematical: the sphere has the minimal surface area to volume ratio of any shape. As a consequence, the spherical shape increases the snowman's longevity: the smaller the surface area, the slower the melting process, and so your snowy friend will stick around longer.
Mathematically perfect snowman proportion
If you go through several websites that offer advice on constructing the perfect snowman, you will see that different sources give different optimal ratios of the consecutive sphere diameters. For instance, the students at Bluefield State College, WV, came up with the 1:2:3 ratio, while Dr. James Hind of Nottingham Trent University proposed the 3:5:8 ratio. So what ratio should you choose?
Mathematically, the most beautiful proportion is the golden ratio (most often denoted by φ) with value, approximately, 1:1.618. This ratio repeatedly appears in nature: in the shape of spiral galaxies, hurricanes, or snail shells, in the proportions of the human body, or in the distribution of flower petals, to mention just a few examples. Therefore, it would make perfect sense to build the perfect snowman according to the golden ratio, right?
There is just a minor problem to overcome. Namely, the exact golden ratio is tough to achieve in practice since φ is an irrational number. So we use approximations, and one of the most popular approximations is via the sequence of Fibonacci numbers:
1, 1, 2, 3, 5, 8, 13, 21, 34...
The idea is to start with two 1's and proceed by getting the subsequent term as the sum of the two preceding terms. As you can see, both 1:2:3 and 3:5:8 ratios come directly from the Fibonacci sequence. The deeper into the Fibonacci sequence you reach, the closer to the golden proportion your snowman will be. How cool is that?
Best snow type for building a snowman
Unless you're building a snowman in Minecraft (we have tools even for this videogame: try our minecraft circle calculator), you definitely need the right kind of snow to build any snow structure, be it a snow person or a snow castle.
You specifically need packing snow, which holds together when delicately compressed. We all know that snow sometimes behaves like powder or sand, making it impossible to build anything. What matters here is the moisture content of the snow, that is, the amount of free water relative to the number of ice crystals. It is this water that acts as adhesive, allowing us to turn snow into a structure. When it comes to the moisture content, scientists classify snow into five categories:
- Dry (0% water);
- Moist (<3% water);
- Wet (between 3% and 8% water);
- Very wet (between 8% and 15% water); and
- Slush (>15% water).
When it comes to snowmen, the optimal water content is around 3%, which translates into using moist or wet snow. How to know if the snow in your yard is just fine? A look at any weather app suffices because snow moisture content depends on the air temperature: the snow is wet/moist around the melting point, that is, at 32°F / 0°C.
There's another way of describing how much water (in terms of volume) is contained in a given volume of snow: it's called snow to liquid ratio or snow to water ratio. Most often the snow to liquid ratio is between 10:1 and 15:1, with the average of about 12:1, which means that melting 12 inches of snow will produce 1 inch of liquid water.
Please remember that the outside temperature give you only a rough estimate of the water content (but good enough for a satisfactory snowman-building experience!). It's because the water content of snow depends on many factors, including the snow formation conditions in the upper atmosphere. That's why it is hard to precisely predict this value without making an actual experiment and melting some snow to see how much water it will produce.