Maximum Height Calculator – Projectile Motion
The maximum height calculator is a tool for finding the maximum vertical position of a launched object in projectile motion. Whether you need the max height formula for an object starting directly off the ground or from some initial elevation – we've got you covered. If you're still wondering how to find the maximum height of a projectile, read the two short paragraphs below, and everything should become clear.
How to find the maximum height of a projectile?
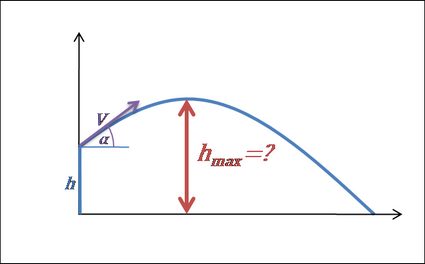
The maximum height of the object is the highest vertical position along its trajectory. The object is flying upwards before reaching the highest point – and it's falling after that point. It means that at the highest point of projectile motion, the vertical velocity is equal to ().
From that equation we can find the time needed to reach the maximum height :
The formula describing vertical distance is:
So, given and , we can join those two equations together:
And what if we launch a projectile from some initial height ? No worries! Apparently, the calculations are a piece of cake – all you need to do is add this initial elevation!
Let's discuss some special cases with changing angle of launch:
- If , then the formula simplifies to:
And the time of flight is the longest.
If, additionally, , then it's the case of free fall, which we detailed at the free fall calculator. Also, you may want to have a look at our even more accurate equivalent – the free fall with air resistance calculator.
- If , then the equation may be written as:
And in that case, the range is maximal if launching from the ground ().
- If , then vertical velocity is equal to (). In this case, we can calculate the horizontal projectile motion. As the sine of is , then the second part of the equation disappears, and we obtain:
The initial height from which we're launching the object is the maximum height in projectile motion.
Maximum height calculator helps you find the answer
Just relax and look how easy-to-use this maximum height calculator is:
-
Choose the velocity of the projectile. Let's type .
-
Enter the angle. Assume we're kicking a ball ⚽ at an angle of .
-
Optionally, type the initial height. In our case, our starting position is the ground, so type in . Can the ball fly over a fence?
-
Here it is – maximum height calculator displayed the answer! It's . So it will not fly over the mentioned barrier – throw it harder or increase the angle to reach your goal.
Just remember that we don't take air resistance into account!
FAQs
How do I find the maximum height of a ball thrown up?
To find the maximum height of a ball thrown up, follow these steps:
- Write down the initial velocity of the ball, v₀.
- Write down the initial height, h₀.
- Replace both in the following formula: h_max = h₀ +(v₀)²/ 2g where g is the acceleration due to gravity, g ~ 9.8 m/s².
What angle has the longest flight time?
90°. You can determine the flight time (t) with the formula t = 2 × V × sin(α) / g. For a given velocity V, this function reaches its maximum value when sin (α) = 1, which occurs at α = 90°.
What factors affect projectile motion?
The main factor that could affect the motion of a projectile is friction. When air resistance is introduced, the friction opposes the direction of movement, decreasing the velocity components of the projectile.
Does the mass of the projectile affect maximum height?
No. The maximum height a projectile can reach after being thrown is only affected by the initial velocity and the launch angle. The mass of the projectile does not affect the maximum height.